Imagine taking a piping hot cup of coffee outside on a crisp autumn morning. As you sip, you notice the warmth gradually fading, the steam dissipating, and the coffee turning lukewarm. This seemingly simple act of cooling is governed by a fundamental principle in physics known as Newton’s Law of Cooling. This law, developed by Sir Isaac Newton, describes the rate at which an object’s temperature changes as it exchanges heat with its surroundings.

Image: www.doovi.com
Learning about Newton’s Law of Cooling isn’t just about understanding how your coffee cools down. It has far-reaching implications in fields ranging from engineering to forensics. Whether you’re designing a cooling system for a computer chip, investigating a crime scene, or simply trying to figure out the optimal time to put your food in the refrigerator, the law provides a powerful framework for predicting and controlling heat transfer.
Delving into the Heart of Newton’s Law of Cooling
Newton’s Law of Cooling states that the rate of heat loss of an object is proportional to the difference between its temperature and the temperature of its surroundings. Essentially, the hotter an object is compared to its environment, the faster it will cool down. This principle can be expressed mathematically as:
dT/dt = -k(T - Ts)Where:
- dT/dt represents the rate of change of temperature of the object over time.
- k is a constant of proportionality that depends on the object’s properties and the environment.
- T is the temperature of the object.
- Ts is the temperature of the surroundings.
The Importance of Understanding the Cooling Constant (k)
The cooling constant, k, is crucial for accurately predicting the temperature change of an object. This constant is influenced by several factors, including:
- Surface Area: Objects with larger surface areas tend to cool faster due to increased exposure to the surrounding environment.
- Material: Different materials have different thermal conductivities, which affect how quickly they transfer heat. For instance, a metal object will generally cool down faster than a wooden object of the same size.
- Convection and Radiation: These heat transfer mechanisms play a role in determining the cooling rate. Convection involves heat transfer through the movement of fluids, while radiation involves heat transfer through electromagnetic waves.
Applications of Newton’s Law of Cooling: Real-World Examples
The power of Newton’s Law of Cooling extends beyond simple coffee cups:
- Criminology: Forensic investigators use this law to estimate the time of death based on the victim’s body temperature. The rate at which a body cools can provide a valuable clue about the time of the crime.
- Food Safety: Understanding the cooling rate of food is crucial for maintaining its safety. Food Safety regulations are based on the principle of keeping perishable items at specific temperature ranges to prevent bacterial growth.
- Industrial Processes: Industries like manufacturing and pharmaceuticals rely heavily on controlled cooling processes. Newton’s Law of Cooling provides the theoretical framework to design efficient cooling systems for products and equipment.
- Climate Science: The law plays a role in understanding how Earth’s atmosphere cools and how greenhouse gases affect global temperatures.
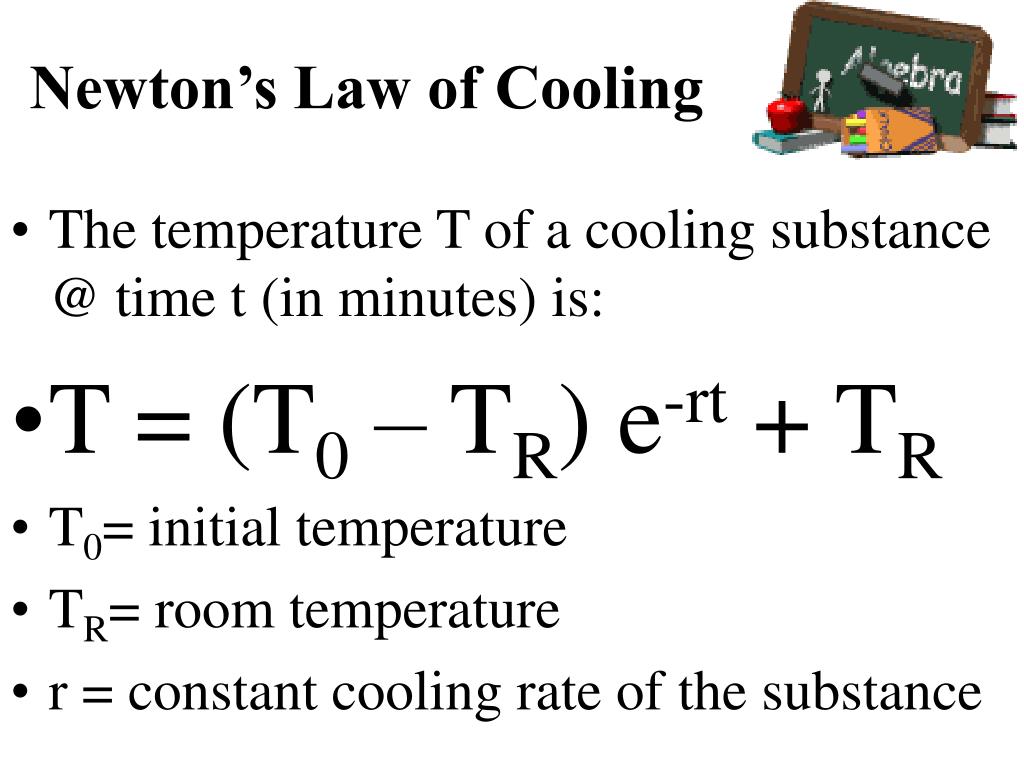
Image: www.slidemake.com
Introducing the Newton’s Law of Cooling Calculator: A Powerful Tool for Analyzing Heat Transfer
The Newton’s Law of Cooling Calculator is a valuable tool for anyone needing to analyze and predict temperature changes. These calculators can be found online, and many are free to use. They typically require the following inputs:
- Initial Temperature of the Object: The temperature of the object at the start of the cooling process.
- Ambient Temperature: The temperature of the surroundings.
- Cooling Constant: The constant of proportionality, which can be determined experimentally or estimated based on the object’s properties and the environment.
- Time Interval: The desired time period for which you want to calculate the temperature.
The calculator then applies Newton’s Law of Cooling equations to predict the temperature of the object at any given time.
Leveraging the Calculator: Practical Tips for Effective Use
To maximize the benefits of a Newton’s Law of Cooling Calculator, here are some tips:
- Accurate Input: Ensure the inputs you provide to the calculator are accurate, as even slight discrepancies can impact the resulting temperature calculations.
- Experimentation: If possible, conduct your own controlled experiment to determine the cooling constant for your specific situation. This will help refine the calculator’s predictions.
- Iterative Process: Don’t solely rely on the initial prediction. Use the results as a starting point and adjust the inputs if necessary to fine-tune the model.
Newton’S Law Of Cooling Calculator
Conclusion: Empowering You to Master Heat Transfer
Newton’s Law of Cooling provides a valuable framework for understanding and predicting the dynamics of heat transfer. By using a calculator and applying the principles of this law, you can gain a comprehensive understanding of how objects cool and how to effectively control these processes. Whether you’re a scientist, engineer, or simply curious about the world around you, the knowledge of Newton’s Law of Cooling empowers you to explore and harness the power of heat transfer.






