Remember those daunting calculus classes? You know, the ones with cryptic symbols like dx/dy scattered across the board? I’ll admit, they were a bit intimidating at first. But once I understood the logic behind them, I began to see them as a powerful tool for understanding the world around us. Derivatives, that’s what dx/dy represents, are the key to understanding the rate of change of a function. They’re used to model everything from the growth of a population to the rise and fall of stock prices.
Image: saesipapicttbr.blogspot.com
From the subtle shift in temperature as you climb a mountain to the rapid acceleration of a car, understanding change is fundamental. Derivatives, represented by that curious notation dx/dy, allow us to quantify and analyze these changes in a precise and elegant way. In this guide, we’ll delve into the world of derivatives, exploring its meaning, applications, and why it holds such importance in various fields.
Unveiling the Secrets of dx/dy
In essence, dx/dy represents the derivative of a function, denoted by y, with respect to a variable x. Imagine a curve traced on a graph, representing the relationship between x and y. The derivative dx/dy essentially measures how much y changes for every small change in x along that curve.
Put simply, dx/dy gives us the slope of the line tangent to the curve at a specific point. It captures the instantaneous rate of change at that very moment. This slope tells us in a precise way how rapidly one variable is changing with respect to the other.
Deciphering the Components: dx and dy
Let’s break down the notation dx/dy into its individual parts. “dx” stands for an infinitely small change in x, and “dy” represents an infinitely small change in y. The “d” symbolizes a differential, implying an infinitesimal change in the respective variables.
Think of it like zooming in infinitely close on a curve. The derivative dx/dy essentially calculates the ratio of these tiny changes, providing us with the instantaneous rate of change at a specific point.
Examples of Derivatives in Action:
Derivatives are not just abstract mathematical concepts — they have real-world applications in various fields:
- Physics: Derivatives are fundamental in physics, where they are used to describe velocity (the rate of change of position) and acceleration (the rate of change of velocity). Imagine tracking the motion of a rocket as it launches. The derivative of the rocket’s position function would give its velocity at any instant in time.
- Economics: Derivatives help economists understand how factors like supply, demand, and prices change over time. For example, the marginal cost of production, which measures the change in cost as the production quantity changes, is found using derivatives.
- Engineering: Derivatives play a crucial role in designing structures and systems. Engineers use them to analyze stress, strain, and other crucial properties of materials, ensuring stability and safety. Imagine building a bridge; derivatives would help optimize its design, ensuring it can withstand various forces.
- Biology: Derivatives are used to model population growth, analyze growth rates of organisms, and understand the spread of diseases. As a simple example, they could be used to predict how a population of bacteria might grow over time.
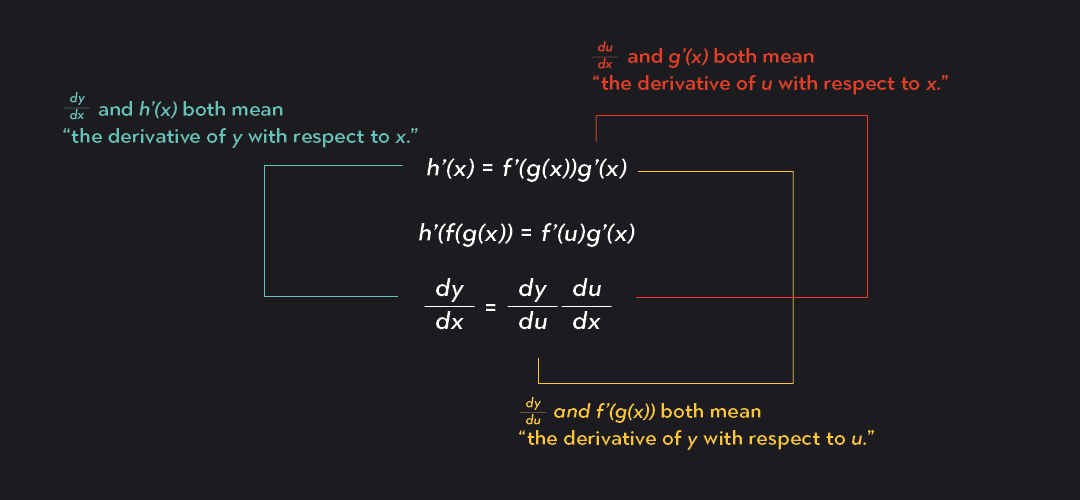
Image: articles.outlier.org
Understanding the Power of Differentiation:
The derivative dx/dy holds immense power because it gives us insights into the rate of change. This ability to quantify change is crucial in many domains.
For example, in physics, knowing the derivative of a position function allows us to calculate the object’s velocity at any given moment. This is important for understanding the motion of objects in a precise way. In economics, derivatives can quantify how marginal utility changes as a consumer consumes more of a product, aiding in understanding consumer behavior.
Staying Ahead: Trends and Developments in Derivatives
The field of derivatives is constantly evolving. Today, researchers explore the use of derivatives in advanced fields such as:
- Artificial Intelligence: Derivatives are being used in machine learning algorithms to optimize parameters and improve model performance. Imagine a self-driving car using derivatives to adjust its steering and braking in real time.
- Data Science: Derivatives play a vital role in data analysis and statistical modeling, helping scientists extract meaningful insights from massive datasets. Imagine analyzing customer data to predict purchasing trends using derivatives and advanced machine learning models.
- Financial Modeling: Derivatives are essential for financial analysts and traders to understand the risks and opportunities associated with investments. They can help in calculating the sensitivity of portfolio values to changes in interest rates or other market factors.
Tips and Expert Advice:
If you’re interested in diving deeper into the world of derivatives, here are some expert tips from my experience:
- Start with the Fundamentals: Gain a strong foundation in calculus, particularly the concepts of limits, continuity, and differentiation. Mastering these basics will make understanding derivatives much easier.
- Visualization is Key: Try to visualize the concepts of derivatives. Use graphs, charts, and real-world examples to help you understand how derivatives relate to change.
- Practice, Practice, Practice: The best way to learn derivatives is through practice. Work through problems, solve examples, and apply the concepts to real-world scenarios.
- Seek Out Resources: Don’t hesitate to explore books, online resources, and tutorials. There are countless materials available that can aid your understanding.
FAQ:
Q: What is the difference between dx/dy and dy/dx?
A: dx/dy and dy/dx are reciprocals of each other. dx/dy represents the derivative of y with respect to x, while dy/dx represents the derivative of x with respect to y. In essence, they provide different perspectives on how one variable changes with respect to the other.
Q: Why are derivatives so important in mathematics?
A: Derivatives are a cornerstone of calculus, allowing us to analyze the rate of change of a function. They provide a powerful tool for understanding not just how things change, but also how fast they change. This is crucial for solving problems in various fields, including physics, engineering, and economics.
Q: How can I learn more about derivatives?
A: There are plenty of resources available for learning more about derivatives. You can start with online tutorials, textbooks, and even interactive platforms. Don’t hesitate to seek the guidance of a teacher or tutor if you need additional help.
What Does Dx/Dy Mean
https://youtube.com/watch?v=SNlKMPHjCwA
Conclusion:
dx/dy represents the derivative of a function, a crucial concept in calculus and a fundamental tool for analyzing rates of change. We explored its definition, application in various fields, and its importance in understanding the world around us.
Are you eager to explore the fascinating world of derivatives further? Let us know in the comments if you have any questions or share your own experiences with derivatives!






